Continued fractions and pi

I suggest the following activity for bright middle-school students who think that they know everything that there is to know about fractions.

The approximation to that is most commonly taught to students is . As I’ll discuss, this is the closest rational number to using a denominator less than . However, it is possible to obtain closer rational approximations to using larger numbers. Indeed, the ancient Chinese mathematicians were superior to the ancient Greeks in this regard, as they developed the approximation

It turns out that this is the best rational approximation to using a denominator less than . In other words, is the best approximation to using a reasonably simple rational number.

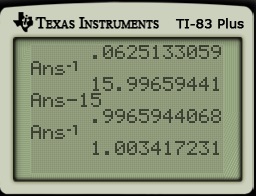
Step 1. To begin, let’s find with a calculator. Then let’s now subtract and then find the inverse.

This calculation has shown that

If we ignore the , we obtain the usual approximation

Step 2. However, there’s no reason to stop with one reciprocal, and this might give us some even better approximations. Let’s subtract from the current denominator and find the reciprocal of the difference.

At this point, we have shown that

If we round the final denominator down to , we obtain the approximation

Step 3. Continuing with the next denominator, we subtract and take the reciprocal again.

At this point, we have shown that

If we round the final denominator down to , we obtain the approximation

Step 4. Let me show one more step.

At this point, we have shown that

If we round the final denominator down to , we (eventually) obtain the approximation

The calculations above are the initial steps in finding the continued fraction representation of . A full treatment of continued fractions is well outside the scope of a single blog post. Instead, I’ll refer the interested reader to the good write-ups at MathWorld (http://mathworld.wolfram.com/ContinuedFraction.html) and Wikipedia (http://en.wikipedia.org/wiki/Continued_fraction) as well as the references therein.

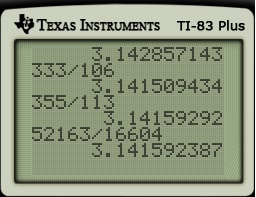
But I would like to point out one important property of the convergents that we found above, which were

All of these fractions are pretty close to , as shown below. (The first decimal below is the result for .)

In fact, these are the first terms in a sequence of best possible rational approximations to $\pi$ up to the given denominator. In other words:

• is the best rational approximation to using a denominator less than $106$. In other words, no integer over will be any closer to than .  No integer over will be any closer to than . And so on, all the way up to denominators of . Small wonder that we usually teach children the approximation .
• Once we reach , the fraction is the best rational approximation to using a denominator less than .
• Then is the best rational approximation to using a denominator less than .

As noted above, the ancient Chinese mathematicians were superior to the ancient Greeks in this regard, as they were able to develop the approximation . For example, Archimedes was able to establish that